What Is a “Return”?
Jul 26, 2021
There’s an old investment joke that goes, “Do investors care more about the return on their investment or the return of their investment?” We want growth plus our original investment back to us, don’t we?
We’re going to focus here on explaining what an investment return is – the growth of capital after it is deployed.
I can’t write about numbers and calculations without quoting Mark Twain, who popularized the saying, “There are three kinds of lies: lies, damned lies, and statistics!” I think he also had a version of the first joke…
GICs
GIC (Guaranteed Investment Certificate) returns are pretty simple to describe and understand, since what they are is usually included right in the investment description. You place a five-year deposit at 4%, and you earn 4% per year for five years.
Annual vs. Compound vs. Total Period Return
In the above example, one could imagine that after five years at 4% per year, the investment would be worth 20% more (5 x 4% = 20%). This is not so – it’s actually worth 21.665% more!
Every year, the 4% annual return is added to the capital for the next year’s growth rate to apply. Year one, the 4% rate applies to the original investment of $100,000, and at the end of the year the investor has $104,000, including $4,000 of growth. The following year, the interest rate of 4% (because you locked in that interest rate for five years) applies to the full $104,000, meaning growth of $4,160 in the second year (4% of $104,000), which is $160 more growth than in the first year.
Over five years, with growth on top of growth on top of growth, the deposit is worth $121,665.29.
One might say they are earning $4,000 per year, but only if they are drawing out the interest annually, and not allowing for the growth to compound.
Now we see that the annual return is 4%, and if allowed to compound (not drawn out every year), will give the investor a total period return over the five years of 21.665%.
Money-Weighted Rate of Return (MWRR) vs. Time-Weighted Rate of Return (TWRR)
In Canada, the standard practice is to use MWRR when calculating returns for individual investors and TWRR when publishing the returns of investment funds.
MWRR takes into account the timing and amounts of additions and withdrawals of the investor, whereas TWRR removes that effect so that the published return can be fairly represented to the public at large – it better measures the manager’s performance without the distorting effects of the investor’s cash flows in and out.
If you had one investment fund only, and made any additions or withdrawals, your rate of return for the year would be different than what the fund published as its return.
Let’s look at an example to see what happens in real life.
What return does Liz earn if she invests $1,000,000 on January 1, makes no withdrawals or additions to her portfolio, and it’s worth $1,100,000 on December 31? That’s easy – it’s 10%.
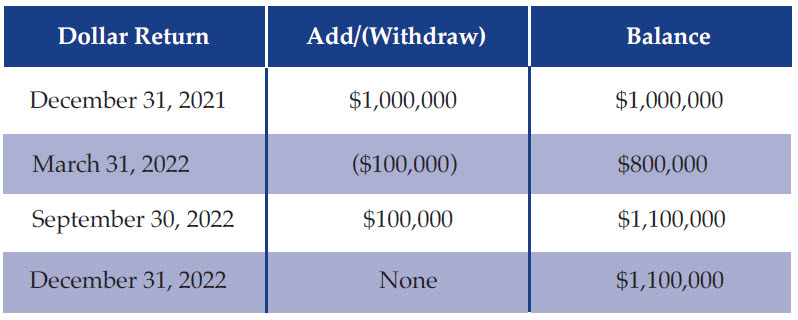
Now we’re going to add a wrinkle. Supposing Liz had made a single portfolio withdrawal of $100,000 on March 31 after the portfolio had already dropped to $900,000, followed by replacing that $100,000 to the portfolio on September 30 when it had grown back up to 1,000,000 in the interim six months. She still ended with $100,000 more than the $1 million (net of deposits and withdrawals) that she invested. What is her return then? Is it 10%?
TWRR will show a rate of return of 12.5% for the year.
A return is calculated from January 1 to March 31 with a drop of $100,000 (-10%), then another from April 1 to September 30 when the portfolio grew $200,000 on the $800,000 left (after the withdrawal) (25%), then another again from October 1 to December 31 when the portfolio did not grow from the $1,100,000 (after the withdrawal had been returned and added to the $1,000,000 value on September 30).
Dollar Return
Because of the differences in calculating percentages, many investors rely on the simplest possible calculation – one that is difficult to misinterpret – how much money did I make? In my example to the left, Liz made $100,000. After all was said and done, her net deposits (additions minus withdrawals) were $1,000,000 and she ended the period with $1,100,000. Simple.
There is no “right” way to calculate a return. These are different methods to achieve the same end, which is to understand how well an investment is performing. Understanding a little more is always better. ■
Business Owners
The most overlooked area of financial planning for business owners and incorporated professionals is the lack of integration between corporate and personal assets. When the majority of your assets are in your corporation you need very specific, specialized and personalized financial advice.
Learn More